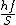Thank you for you reply,That point in Cartesian coordinates system would be at 0 on the x-axis, 0 on the y-axis and 1 on the z-axis.
Your use of the term vector doesn't make sense in the context you presented.
There are many ways to represent a vector. You could say that you have a vector a, defined as a = [0, 0, 1], which would be a vector of length 1 in the z direction. This particular vector is also known as a unit vector in the z direction; $$ \hat{k}$$
If I wanted to displace an object a = [0, 0, 1], one space to the right , a=[1,0,1] , how would I show this?
Would we put Δa = [0, 0, 1]= a=[1,0,1]?
Last edited: