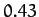exchemist
Valued Senior Member
Nice explanation.Simplified scenario:
Probe is launched from Earth into elliptical orbit that reaches Jupiter orbit. It arrives moving at a velocity less than Jupiter's but ahead of it. Let's say that it is moving at 8 km/sec or 5km/sec slower than Jupiter relative to the Sun.* This means it starts with a 5/km sec velocity in Jupiter's direction.
Jupiter's gravity pulls it towards it even faster, and it goes into a parabolic orbit around Jupiter.** This means it falls in towards Jupiter, whips around it and heads back the way it came from. It returns to its starting distance from Jupiter, with the same difference in speed 5 km/sec, but heading away from Jupiter in the same direction as Jupiter is moving away relative to the Sun. So before it started falling towards Jupiter, it was moving at 13-5 = 8km/sec relative to the Sun, and after whipping around Jupiter, it is moving at 13+5 = 18 km/sec relative to the Sun. The flip side of this is that Jupiter also "falls'' toward the probe, and performs a "mirrored" trajectory, which ends up subtracting a bit of its orbital velocity relative to the Sun. There is a momentum exchange between Jupiter and the Probe. But since Jupiter out masses the probe by so much, the change in Jupiter's velocity is infinitesimal.
* These values are just for illustration purposes and are not meant to represent the real numbers involved in an actual encounter.
** Again, over simplified. The actual trajectory would be hyperbolic, but this doesn't change the basic principle.
In essence, it is like an elastic collision where one object comes from behind another slower object, bumps into it, increasing the other object's velocity while giving up some of its own. Just instead of physical contact, gravitational interaction provides the means of momentum exchange.