Write4U
Valued Senior Member
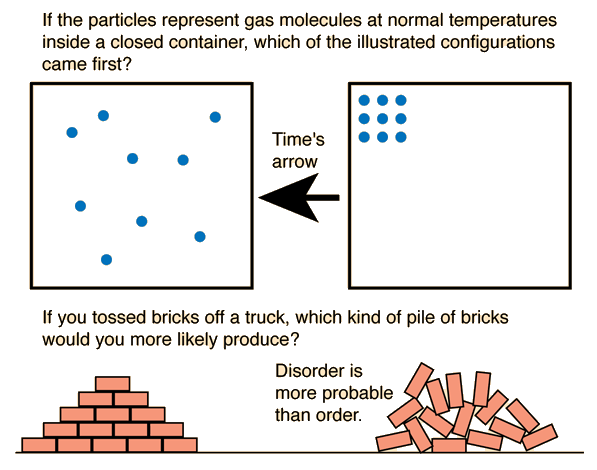
Spacetime IS the mathematical physicality (geometry) which emerged from Chaos.Why should space exist , mathematically ? Devoid of physicality .
I think you have mixed the terms "organization" with "complexity".Michael said;
It created the perfect organisation of stuff by physics because it could not be in any other arrangement at that moment
Last edited: