Didn't I say that earlier?The gravitational field affects the propagation of light. LIGO is the best proof.
Spacetime geometry is the gravitational field, and light follows geodesics in curved spacetime that we see as gravity.
Didn't I say that earlier?The gravitational field affects the propagation of light. LIGO is the best proof.
Yes, so the assumption that the speed of light is constant is wrong. Light is only held by the gravitational field of the earth, and the speed of light measured anywhere on the earth is constant.Didn't I say that earlier?
Spacetime geometry is the gravitational field, and light follows geodesics in curved spacetime that we see as gravity.
Lol, sorry I tagged it to your post.While I don't particularly disagree with what you posted, it seems irrelevant as a response to my post, which involves no light beams or accelerating observers or anything. It was a trivial question of: If two guys are a each a mile from the store in different directions and walking at the same pace towards it, they'll get there at the same time.
Oh, it is pertinent to the general discussion. That's why SR (that makes the constant light speed premise) only holds for the special case of uniform gravitational fields.Lol, sorry I tagged it to your post.
I just thought Einstein's "man in the box" might be pertinent to the discussion of gravitational relativity (refraction?) in general.
Oh, it is pertinent to the general discussion. That's why SR (that makes the constant light speed premise) only holds for the special case of uniform gravitational fields.
It's well known that light speed at c is only a locally measured constant, and hence why light beamed to the moon and reflected back does the round trip in less time than the total distance/c. Not much faster, but measurably so.
Yes, refraction is responsible for some of the bending of light around an object, but not all of it, so an explanation based purely on refraction can be falsified.
No, the speed of light is constant, any variation as measured from an outside FoR, simply allows for a longer "curved"path it has travelled...you know, geodesics.Yes, so the assumption that the speed of light is constant is wrong.
I'm happy to meet you to, and I'm not a physicist, but I still say you are wrong.
That's confusing to put it mildly. but the speed of light ''on" Earth, is the same as it is anywhere else..."c"Light on the earth is held by a gravitational field, and the speed of light measured anywhere on the surface of the earth is constant, the same as it is in space. The speed of light is superimposed on the speed of the gravitational field (that is, the speed of the earth's revolution around the sun).
If the light is aimed at the center of the planet, is the geodesic a straight line or a curve? A geodesic is a trajectory you see. The reason for this trajectory is the non-uniformity of the gravitational field.No, the speed of light is constant, any variation as measured from an outside FoR, simply allows for a longer "curved"path it has travelled...you know, geodesics.
It's hard to illustrate a 3D/4D concept in 2D....but that is a good question. A geodesic simply put, is the shortest path between two points or observers. I would say a photon directed at the center of a planet would like the defintion of a geodesic, follow the shortest path.If the light is aimed at the center of the planet, is the geodesic a straight line or a curve? A geodesic is a trajectory you see. The reason for this trajectory is the non-uniformity of the gravitational field.

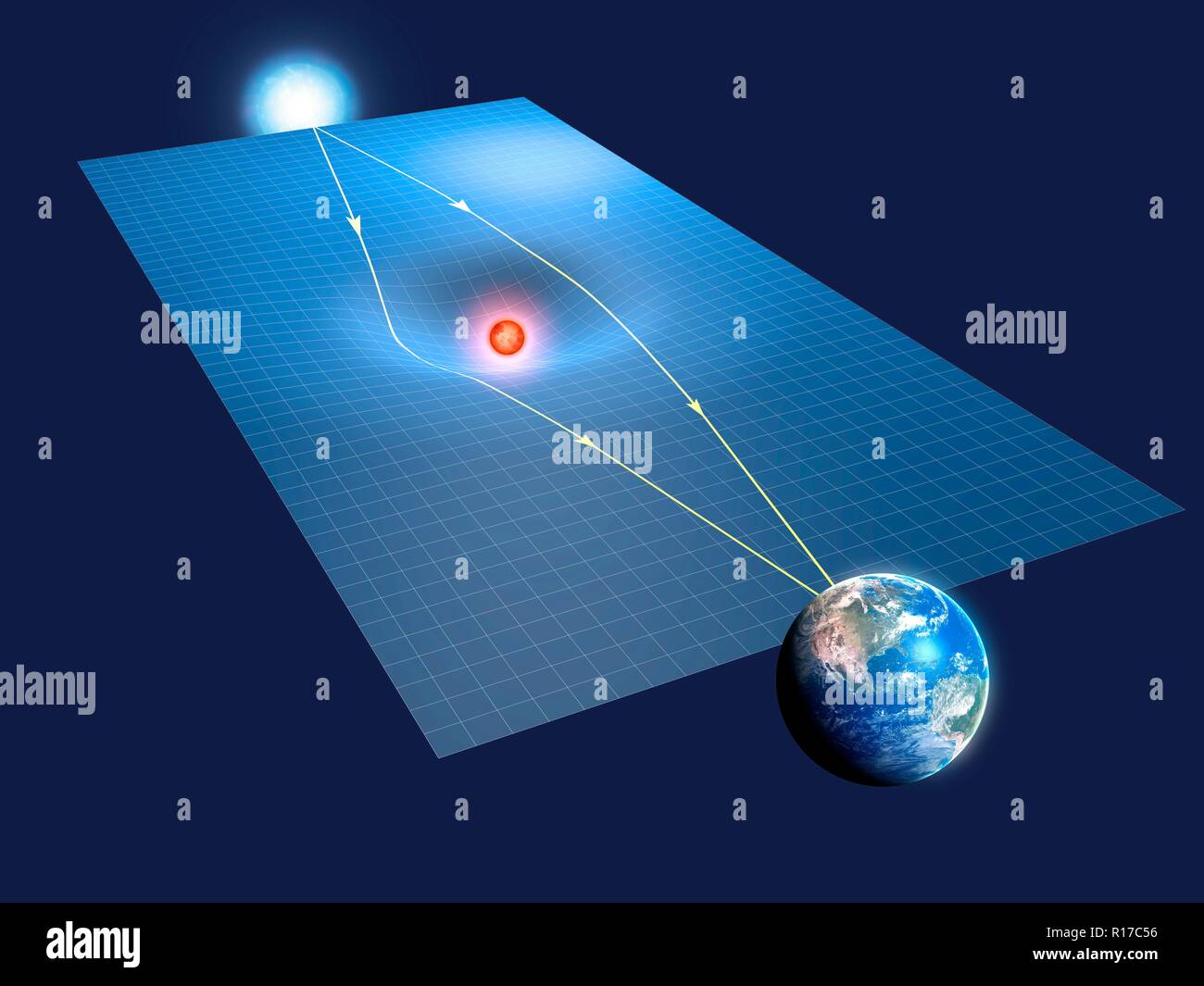

https://photos.app.goo.gl/jDgewZHgjeCPr4jG9https://en.wikipedia.org/wiki/Geodesics_in_general_relativity
In general relativity, a geodesic generalizes the notion of a "straight line" to curved spacetime. Importantly, the world line of a particle free from all external, non-gravitational forces is a particular type of geodesic. In other words, a freely moving or falling particle always moves along a geodesic.
In general relativity, gravity can be regarded as not a force but a consequence of a curved spacetime geometry where the source of curvature is the stress–energy tensor (representing matter, for instance). Thus, for example, the path of a planet orbiting a star is the projection of a geodesic of the curved four-dimensional (4-D) spacetime geometry around the star onto three-dimensional (3-D) space.
Have you ever thought that it would be easier to analyze this process with a moving gravitational field, and Newton's classical mechanics can calculate the planetary trajectory. This trajectory is exactly the geodesic described by general relativity. Although this deviation will be small relative to the stationary gravitational field, it is sufficient to explain Mercury's precession.Is it fair to say that in theory the shortest path is a straight line, but in a curved spacetime geodesic there is no theoretical straight line possible, the light is already following a straight line along the geodesic. In reality no other path is possible?
But we are talking about Light, not about massive objects. The SOL in a vacuum is a constant.Have you ever thought that it would be easier to analyze this process with a moving gravitational field, and Newton's classical mechanics can calculate the planetary trajectory. This trajectory is exactly the geodesic described by general relativity. Although this deviation will be small relative to the stationary gravitational field, it is sufficient to explain Mercury's precession.
https://en.wikipedia.org/wiki/Theory_of_relativitySpecial relativity
The laws of physics are the same for all observers in any inertial frame of reference relative to one another (principle of relativity). The speed of light in a vacuum is the same for all observers, regardless of their relative motion or of the motion of the light source.
The light is similar, I described it in # post 253.But we are talking about Light, not about massive objects. The SOL in a vacuum is a constant.
If you run up an escalator that is moving @ SOL, you do not get to the top any faster than if you stand still...
Well, obviously light does not behave in a gravitational field as is illustrated. That pertains to massive objects only, which are subject to the gravitational pull between two massive objects.The light is similar, I described it in # post 253.
If you return to Newtonian mechanics, your scientific path will be smooth.Well, obviously light does not behave in a gravitational field as is illustrated. That pertains to massive objects only, which are subject to the gravitational pull between two massive objects.
https://photos.app.goo.gl/LbJoTK2q5dX11TBe7
There are usually two reasons that can cause the object's motion path to bend. One is the velocity caused by the force in the vertical direction, and the other is the refraction caused by the unevenness of the medium or the unevenness of the field. Then the bending of the light is probably caused by the latter. The sun is surrounded by the gravitational field. The gravitational field is stronger as it goes inside. When light passes through the gravitational field of the sun, the light is bent similarly to refraction due to the unevenness of the gravitational field. The curvature of this path is different from the curvature caused by the direct action of gravity on an object. Therefore, it is not appropriate to calculate the bending angle of light using the classic flat throw theory.
https://photos.app.goo.gl/fcHk2UjEQufwdmw1A
This is because the theory of flat throw thinks that there is a co-directional acceleration process under the pull of gravity at the beginning, before the acceleration caused by gravity becomes negative, the light will be farther away from the sun and the influence of gravity will be smaller. The deflection angle will also be smaller. This is the reason why the angle of light deflection calculated by the classic flat throw theory is too small. Newtonian mechanics is not wrong, but this time it was not used correctly.
The rotation of the earth does not affect the strength of the gravity field. We should consider the revolution of the earth around the sun, and the gravitational field moves with the earth. The data we calculated are basically consistent with the experimental observation data given by Janus. But Janus thinks that the gravitational field of the earth is too weak to affect the bending of light, which is wrong.
Light deflected by just 0.0006 arc-seconds =1.667*10^-7 degree. This is the observation data given by Janus.
The earth's angular speed : 360/365*24*60*60 = 1.1415*10^- 5 degree/s.This is my calculated angular speed of the Earth's revolution around the sun.
If the time of light passing through the earth is 0.01 seconds, about 3000 km,the angle of rotation of the earth is 1.1415*10^- 7 degree. It can't be ignored!
I am out of my depth here, but it seems to me that a photon's mass is acquired by its momentum but that this kinetic mass is not subject to gravitational pull, but only restricted to follow the geodesics of the warped spacetime itself, but never gets caught in a gravitational well to form an orbit.
This might possibly occur in a Black Hole where the spacetime geodesics themselves fold into themselves?
Don't ask me to prove any of this....
All light (and any other inertial object) follows a geodesic through spacetime, per GR. Where it is aimed has nothing to do with it. A geodesic is always a straight line in the coordinate space in which it is defined. That means if you toss a paper wad into the trash across the room, and discounting air friction, the wad of paper traces a straight line through spacetime. This is true even for the slow, hi-arced shot and the faster attempt that follows a different path through space, but both straight lines through spacetime.If the light is aimed at the center of the planet, is the geodesic a straight line or a curve?
