Pretty scathing, huh?
Well I don't know Tegmark and from the small sample of the sample of the scathing review I am unlikely to look any further in depth
Pretty scathing, huh?
Then stop referring to authority to debunk Tegmark.Noooooo I showed you a example of my magical thinking. The type of magical thinking I assess Tegmark engages in
Please stop with the constant reference to authority
Nothing you have shown me debunks Tegmark's mathematical universe. Talk about LEVELS does not have any bearing on the concept of a mathematical universe.Believe or not NOT EVERYTHING NEEDS A SCIENTIFIC REFERENCE










In logic, mathematics and linguistics, And (∧) is the truth-functional operator of logical conjunction; the and of a set of operands is true if and only if all of its operands are true. The logical connective that represents this operator is typically written as ∧ or ⋅ .[1][2][3]



Beyond logic, the term "conjunction" also refers to similar concepts in other fields:
- In natural language, the coordinating conjunction "and".
- In programming languages, the short-circuit and control structure.
- In set theory, intersection.
- In lattice theory, logical conjunction (greatest lower bound).
- In predicate logic, universal quantification.
Logical conjunction is an operation on two logical values, typically the values of two propositions, that produces a value of true if and only if both of its operands are true.[2][3]
https://en.wikipedia.org/wiki/Logical_conjunctionThe conjunctive identity is true, which is to say that AND-ing an expression with true will never change the value of the expression. In keeping with the concept of vacuous truth, when conjunction is defined as an operator or function of arbitrary arity, the empty conjunction (AND-ing over an empty set of operands) is often defined as having the result true.
Logical conjunction
Definition
Truth table
Logic gate
Normal forms
Disjunctive
Conjunctive
Zhegalkin polynomial
Post's lattices
0-preserving yes
1-preserving yes
Monotone no
Affine no
Venn diagram of
is true if and only if is true and
is true and is true.
is true.
An operand of a conjunction is a conjunct.
Ok then, if that's all it is to you......Blah blah blah


I know, the abstract mathematical world is truly amazing. But then Mathematics is not a Conscious Intelligence or an Artificial Intelligence, but a Quasi-intelligent functional property of spacetime geometry.Blah blah with pictures


In physics, spacetime is any mathematical model which fuses the three dimensions of space and the one dimension of time into a single four-dimensional manifold. Spacetime diagrams can be used to visualize relativistic effects, such as why different observers perceive where and when events occur differently.
Until the 20th century, it was assumed that the 3-dimensional geometry of the universe (its spatial expression in terms of coordinates, distances, and directions) was independent of one-dimensional time. However, in 1905, Albert Einstein based a work on special relativity on two postulates:
The laws of physics are invariant (i.e., identical) in all inertial systems (i.e., non-accelerating frames of reference)
The speed of light in a vacuum is the same for all observers, regardless of the motion of the light source.
The logical consequence of taking these postulates together is the inseparable joining together of the four dimensions—hitherto assumed as independent—of space and time.
.......Many counterintuitive consequences emerge: in addition to being independent of the motion of the light source, the speed of light is of constant magnitude regardless of the frame of reference in which it is measured; the distances and even temporal ordering of pairs of events change when measured in different inertial frames of reference (this is the relativity of simultaneity); and the linear additivity of velocities no longer holds true
In 1908, Hermann Minkowski—once one of the math professors of a young Einstein in Zürich—presented a geometric interpretation of special relativity that fused time and the three spatial dimensions of space into a single four-dimensional continuum now known as Minkowski space. A key feature of this interpretation is the formal definition of the spacetime interval. Although measurements of distance and time between events differ for measurements made in different reference frames, the spacetime interval is independent of the inertial frame of reference in which they are recorded.[1]
https://en.wikipedia.org/wiki/SpacetimeMinkowski's geometric interpretation of relativity was to prove vital to Einstein's development of his 1915 general theory of relativity, wherein he showed how mass and energy curve flat spacetime into a pseudo-Riemannian manifold.
In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a lake.
In physics, a dynamical system is described as a "particle or ensemble of particles whose state varies over time and thus obeys differential equations involving time derivatives."[3] In order to make a prediction about the system's future behavior, an analytical solution of such equations or their integration over time through computer simulation is realized.
https://en.wikipedia.org/wiki/Dynamical_systemDynamical systems are a fundamental part of chaos theory, logistic map dynamics, bifurcation theory, the self-assembly and self-organization processes, and the edge of chaos concept.
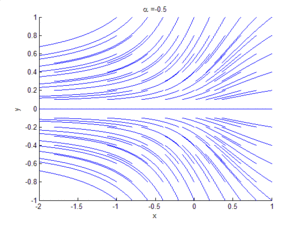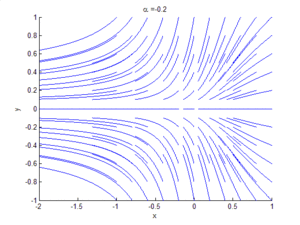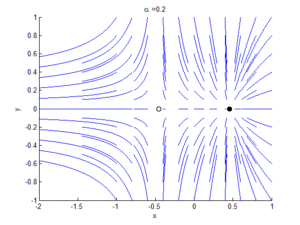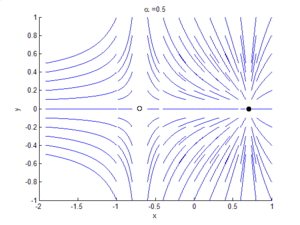
Bifurcation theory is the mathematical study of changes in the qualitative or topological structure of a given family, such as the integral curves of a family of vector fields, and the solutions of a family of differential equations.
Most commonly applied to the mathematical study of dynamical systems, a bifurcation occurs when a small smooth change made to the parameter values (the bifurcation parameters) of a system causes a sudden 'qualitative' or topological change in its behavior.[1]
https://en.wikipedia.org/wiki/Bifurcation_theoryBifurcations occur in both continuous systems (described by ODEs, DDEs or PDEs) and discrete systems (described by maps). The name "bifurcation" was first introduced by Henri Poincaré in 1885 in the first paper in mathematics showing such a behavior.[2] Henri Poincaré also later named various types of stationary points and classified them.
In physics, a dimensionless physical constant is a physical constant that is dimensionless, i.e. a pure number having no units attached and having a numerical value that is independent of whatever system of units may be used.[1]:525 For example, if one considers one particular airfoil, the Reynolds number value of the laminar–turbulent transition is one relevant dimensionless physical constant of the problem. However, it is strictly related to the particular problem: for example, it is related to the airfoil being considered and also to the type of fluid in which it moves.
On the other hand, the term fundamental physical constant is used to refer to some universal dimensionless constants. Perhaps the best-known example is the fine-structure constant, α, which has an approximate value of 1⁄137.036.[2]:367
https://en.wikipedia.org/wiki/Dimensionless_physical_constantHowever, the term fundamental physical constant has been sometimes used to refer to certain universal dimensioned physical constants, such as the speed of light c, vacuum permittivity ε0, Planck constant h, and the gravitational constant G, that appear in the most basic theories of physics.[9][10][11][12] NIST[9] and CODATA[13] sometimes used the term in this way in the past.
The common notation t = 0 simply means "time equals zero." It expresses the radical and fundamental conclusion of standard Big Bang cosmology. It recognizes the connection between space and time by implying that the Big Bang did not occur in time but that time began with the Big Bang singularity.
For many theological thinkers, the scientific articulation of t = 0 has significant implications for understanding creation, particularly the idea of a creation out of nothing (creatio ex nihilo ).
No one claims that the universe is a Living thing!
Write4U said: ↑
No one claims that the universe is a Living thing!
Yes, but needs not be alive to act (behave) in a quasi-intelligent manner.Your claim it is quasi intelligent
See above.Which quasi?
Yes, stuff behaving in a regular (measurable) order, "AS IF" it were intelligent. (The original basis for Theism)Dare I say us Minions are intelligent, but when talking about the Universe as the Universe we normally mean "a whole lot of stuff other than us"
But now we are talking about degrees of intelligent behaviors. i.e. (to name a few);That whole lot of stuff other than us is not within a Planck distance of being intelligent
I agree, universal mathematics are not arbitrary, they are strictly relational. i.e. Constants (differential equations).While in the area - it is not a arbitrary made up system (maths) Universe either.

I might add: Chemistry, Minarology,A mathematical constant is a key number whose value is fixed by an unambiguous definition, often referred to by a symbol (e.g., an alphabet letter), or by mathematicians' names to facilitate using it across multiple mathematical problems.[1][2] Constants arise in many areas of mathematics, with constants such as e and π occurring in such diverse contexts as geometry, number theory, and calculus.
https://en.wikipedia.org/wiki/Mathematical_constantArchimedes' constant...................................... π
The imaginary unit.......................................... i
Euler's number............................................... e.
Pythagoras' constant.......................................√2.
The Feigenbaum constants............................. α and δ
Apéry's constant............................................. ζ(3)
The golden ratio.............................................. φ
The Euler–Mascheroni constant......................γ
More items...

in human symbolic terms.
https://en.wikipedia.org/wiki/Function_(mathematics)#If the function is called f, this relation is denoted by y = f(x) (which reads "f of x"), where the element x is the argument or input of the function, and y is the value of the function, the output, or the image of x by f.[2]
The symbol that is used for representing the input is the variable of the function (e.g., f is a function of the variable x).[3]
Write4U said: ↑
No one claims that the universe is a Living thing!
So does an aquarium...But it has life with in it .
river said: ↑
But it has life with in it .
An atom is the smallest unit of matter that retains all of the chemical properties of an element. Atoms combine to form molecules, which then interact to form solids, gases, or liquids. For example, water is composed of hydrogen and oxygen atoms that have combined to form water molecules. Many biological processes are devoted to breaking down molecules into their component atoms so they can be reassembled into a more useful molecule.
Nice poems.
But;
No one claims that the universe is a living thing!
Repeat;
No one claims that the universe is a Living thing!
Repeat;
No one claims that the universe is a Living thing!
Remember;
No one claims that the universe is a Living thing!
"Quasi Intelligent" does NOT mean a "Living Thing!
Remember;
No one claims that the universe is a Living thing!
If you think someone is claiming that the Universe is a Living thing, you are wrong.......
Love the poems.....
And how do you keep stuff apart? It's not all the same stuff, is it? There is even stuff within stuff. How does it do that?
Is it magical beyond our cognition and understanding ? Or are we able to analyze the mathematical data by which different stuffs may be relationally connected. There is nothing magical about the concept of a mathematically describable relational interaction of fundamental stuffs (values), by means of a symbolic translation of a dynamical but orderly reality and how these interactive properties can be applied to human survival mechanisms.
We do not describe Global warming as "stuff happening". Knowledge of the mathematics in a probabilistic but deterministic world may come in handy some day!
We may not necessarily know "when", but knowing (calculating) "what" may happen in the future is a survival strategy in an "grander" evolutionary context......
The Universe is not an unknowable mystery! It is a physical object and can be abstractly analyzed as to inherent mathematically calculable potentials and probable behaviors.
Else we might as well believe in God, the anthropomorphized imaginary, unexplainable but benevolent spiritual equivalent (=) of mathematically expressed Potential ("That which may become reality"), a potential future probability based on patterns with measurable relational values and mathematical (algebraic) functions.
