nebel:
The situation referred to was not about a planet, but a shrinking globe, planet or star if you will. so: In the process of compression, in the volume defined by the green lines, -the space that is no longer part of the globe,- stronger gravity on the outside (red) has replaced the weaker , (sloping blue line) formerly inner gravity.
I can't see the image you are referring to. Perhaps you tried to embed it in your post, but it didn't work. I can't tell what you're talking about here.
Since the inner and outer gravity strength near the surface is roughly equal, but the space outside is vastly greater, there is much more gravity in total outside, stretching even to infinity, whereas the already smaller inner gradient dives to zero quickly.
I still can't work out what you mean when you say there is "much more gravity" somewhere. It sounds like you think gravity is like a substance that varies in density, or something like that. Gravity is a force (let's go with the Newtonian picture unless we find we need General Relativity). We can meaningfully talk about the acceleration due to gravity at any point in space, but talking about "much more gravity" seems meaningless to me, unless you simply mean the acceleration due to gravity is greater. It sounds to me like this is
not what you mean, though. So, I'll ask again: what do you mean when you say there is "much more gravity" in one place, compared to another? Be precise.
So , in any contracting entity,
A weaker, and smaller gravity field in the interior will be replaced by a stronger and larger gravity field on the outside surface, in addition to the residue gravity always remaining to infinity.(blue curve).
It is possible that all you are saying here is that as a spherical mass is compressed, the acceleration due to gravity at its surface increases. That is correct. Is that all you're saying?
Gravity that clearly is a feature, kind of emanating or projecting outwardly , never inwardly from a mass
That's false. The gravitational field from a mass projects in all directions. Every bit of mass attracts every other bit of mass, at the fundamental level.
To understand the situation you have to treat the interior of a globe as a stacked series of shells, and irregular masses as more or less close approximations.
Irregularly-shaped masses are seldom close approximations to spheres.
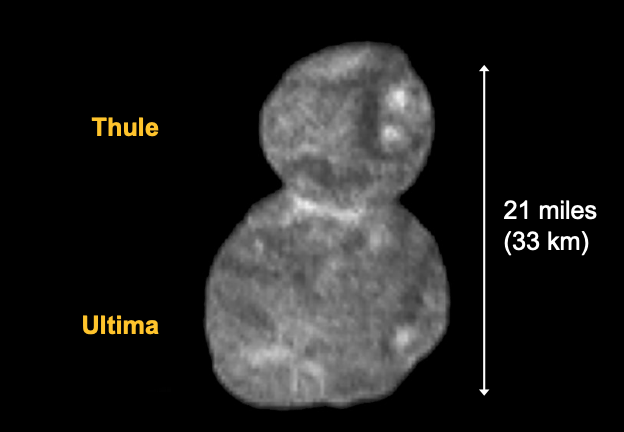
I am using this image from BBC science to illustrate my Inside / Outside & developing gravity understanding. In the image,
The object's center of gravity, and axis of rotation, lies about on a horizontal line level with the (33 km) writing.
I don't know how you know this. Assuming approximately uniform density, that looks reasonable, though.
That central point is surrounded by equal masses in all directions, pulling with equal force
No, because the object is clearly not spherical. There is a distribution of mass at different distances from the centre of mass of the object. Mass close to the centre of mass produces a stronger gravitational field there than mass that is further away from the centre of mass. Overall, though, all the fields from the individual mass elements add up (as vectors) to create zero net acceleration due to gravity at the centre of mass.
However before this entity came together into that shape, there were strong surface gravity fields projecting out from both globes. Those fields, at contact, were eliminated and a new total gravitational field now surrounds Thule Ultima[tely].
The fields of the two "pieces" of the combined object simply add as vectors everywhere to produce a net field. Nothing about the fields of the two pieces taken individually changes, regardless of whether the pieces are far apart or joined as we see in the picture. The net gravitational field of the joined object is simply the superposition of the individual fields of the two pieces.
There's no "totally new gravitational field" at work here - just a combination of the two pre-existing fields from the masses of the two pieces.
When two soap bubbles merge in similar manner, you can actually watch the surface tension migrate to the common outside
Surface tension is not the same as gravity. It is, as the label says, a surface or boundary phenomenon. Gravity is a bulk phenomenon.
Gravity too is an outside, strongly surface phenomenon.
This is quite wrong, I'm afraid.